Remember trying to understand how quickly money grows in a savings account? Or how a viral video can spread like wildfire across the internet? These scenarios are perfect examples of exponential growth, a concept explored in depth in Unit 6 of many math courses. But, what if you’re struggling with the homework problems and wish you had a magic solution? That’s where a unit 6 exponents and exponential functions answer key PDF might come in handy.
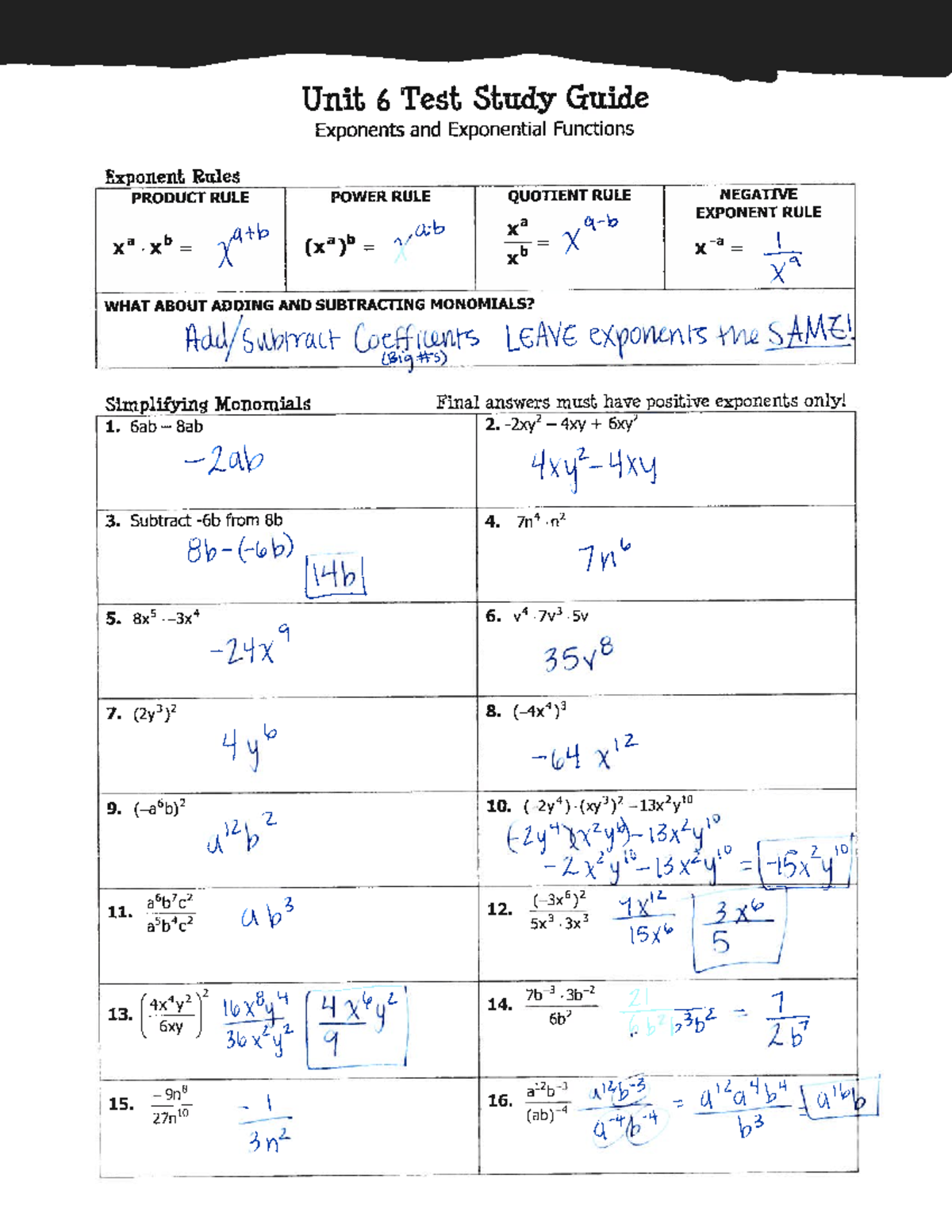
Image: www.studocu.com
Don’t worry! We’re not advocating for cheating. Answer keys play a valuable role in learning. They can help you check your own work, identify your weak areas, and understand the thought process behind solving exponential problems. Even if you’re a pro at exponents, a well-crafted answer key can be a helpful tool for practicing and reinforcing your knowledge. Let’s dive into the world of exponents and exponential functions and understand why having an answer key in your arsenal can be a game-changer!
Understanding the Importance of Unit 6 Exponents & Exponential Functions
Unit 6 in many math courses is dedicated to the fascinating realm of exponents and exponential functions. This unit delves into the concept of repeated multiplication, where a base number is multiplied by itself a certain number of times. For example, 2 to the power of 3 (written as 2^3) represents 2 multiplied by itself three times: 2 * 2 * 2 = 8.
But why is this unit so important? Exponential functions are found everywhere in our daily lives. They model population growth, radioactive decay, compound interest, and much more. Understanding them unlocks the ability to analyze, predict, and solve problems related to these phenomena.
Delving into Unit 6: Exponents and Exponential Functions
Exponential Functions: The Foundation of Growth
Exponential functions take the form y = a * b^x, where:
- a is the initial value (the value of y when x = 0)
- b is the base (the number being multiplied by itself)
- x is the exponent (the number of times the base is multiplied by itself)
The value of ‘b’ plays a crucial role in determining the shape of the graph. If ‘b’ is greater than 1, the function represents exponential growth. Conversely, if ‘b’ is between 0 and 1, it represents exponential decay. The higher the value of ‘b,’ the steeper the curve of the exponential function.

Image: worksheetcampuskrueger.z21.web.core.windows.net
Applications of Exponents & Exponential Functions
Exponents and exponential functions have a vast array of applications, including:
- Finance: Understanding compound interest and the growth of investments
- Biology: Modeling population growth and radioactive decay
- Computer Science: Analyzing the complexity of algorithms and the growth of data storage
- Physics: Describing radioactive decay and exponential growth in reactions
- Technology: Modeling the spread of viruses and the growth of social media networks
Solving Exponential Equations
Solving exponential equations involves finding the value of the unknown exponent. This can be achieved using several techniques, including:
- Isolating the exponent: This usually involves using logarithms
- Graphing: Visualizing the relationship between the exponent and the resulting value
- Trial and error: Trying different values of the exponent until you find the correct one
Exponential Growth and Decay
Understanding Exponential Growth and Decay is crucial because these concepts have real-world applications across different fields.
- Exponential Growth: This occurs when a quantity increases at an accelerating rate, where the rate of growth is proportional to the current size of the quantity.
- Exponential Decay: This occurs when a quantity decreases at a decreasing rate, where the rate of decrease is proportional to the current size of the quantity.
Examples of Exponential Growth include:
- Population Growth: The size of a population can increase exponentially, especially in resource-rich environments.
- The Spread of Infectious Diseases: In the early stages of an outbreak, the number of infected individuals can rise exponentially due to close contact with others.
Examples of Exponential Decay include:
- Radioactive Decay: Radioactive substances decay exponentially, meaning that their activity decreases over time at a rate proportional to the amount of radioactive material present.
- The Rate of Cooling of a Hot Object: Objects cool down at a decreasing rate, with the rate of cooling being proportional to the temperature difference between the object and its surroundings.
Mastering Unit 6 with Answer Key PDFs
Here’s where an answer key PDF can be a real asset in your learning journey. Think of it as a study buddy! It can provide:
- Instant feedback: Get immediate confirmation on whether your solutions are accurate.
- Step-by-step solutions: Unravel the logic behind solving complex exponential problems. Learn the process, not just the answer.
- Clarity on challenging concepts: Understand the rationale behind different approaches to solving exponential equations.
Using a Unit 6 Answer Key PDF Effectively
To get the most out of your answer key, follow these tips:
- Attempt each problem first: Don’t just jump to the answer key. Try solving each problem on your own. This way, you gain a deeper understanding of the concepts.
- Review the answer key only when you’re stuck: Use the answer key as a guide, not a crutch. If you’re struggling with a particular problem, then look at the solution. But make sure you understand the steps involved before moving on.
- Go back and repeat the problem: Once you’ve understood the solution provided in the answer key, try solving the problem again on your own. This helps strengthen your understanding.
- Focus on the process, not just the answer: Pay close attention to the reasoning and steps involved in arriving at the solution. Understanding the process is more important than memorizing the answer.
FAQs about Unit 6 Exponents and Exponential Functions
Here’s a breakdown of some commonly asked questions about Unit 6:
Q: What are the key concepts covered in Unit 6?
A: Unit 6 typically covers definitions of exponents and exponential functions, graphing exponential functions, solving exponential equations, understanding exponential growth and decay, and applying exponential functions to real-world scenarios.
Q: Why are exponents and exponential functions so important?
A: The concepts of exponents and exponential functions are fundamental for understanding growth and decay in various fields like finance, biology, computer science, and physics. They allow us to model and analyze how things change over time.
Q: How can I improve my understanding of exponents and exponential functions?
A: Practice solving problems, review examples provided in textbooks or online resources, watch video tutorials, ask for help from teachers or classmates, and utilize online tools for visualizing exponential functions.
Q: Where can I find a unit 6 exponents and exponential functions answer key PDF?
A: The best place to start is by checking your textbook website or contacting your teacher. You can also try searching online for “unit 6 exponents and exponential functions answer key PDF,” but ensure you use a reliable source.
Unit 6 Exponents And Exponential Functions Answer Key Pdf
https://youtube.com/watch?v=8Y1P6h9Jnws
Conclusion: Empowering Yourself with Exponential Knowledge
Mastering Unit 6 on exponents and exponential functions can truly enhance your understanding of the world around us. Remember, an answer key can be a valuable tool, but it’s essential to use it strategically to learn and solidify your understanding. By actively engaging with the material, practicing, and using tools like answer keys, you can build a strong foundation in exponents and exponential functions.
Are you ready to conquer Unit 6 and unlock the power of exponential growth? Let us know in the comments below!






